Challenging Geometry Puzzle: Problem 1566. Share your solution by posting it in the comment box provided.
Audience: Mathematics Education - K-12 Schools, Honors Geometry, and College Level.
Gain comprehensive insights! Click below to reveal the complete details.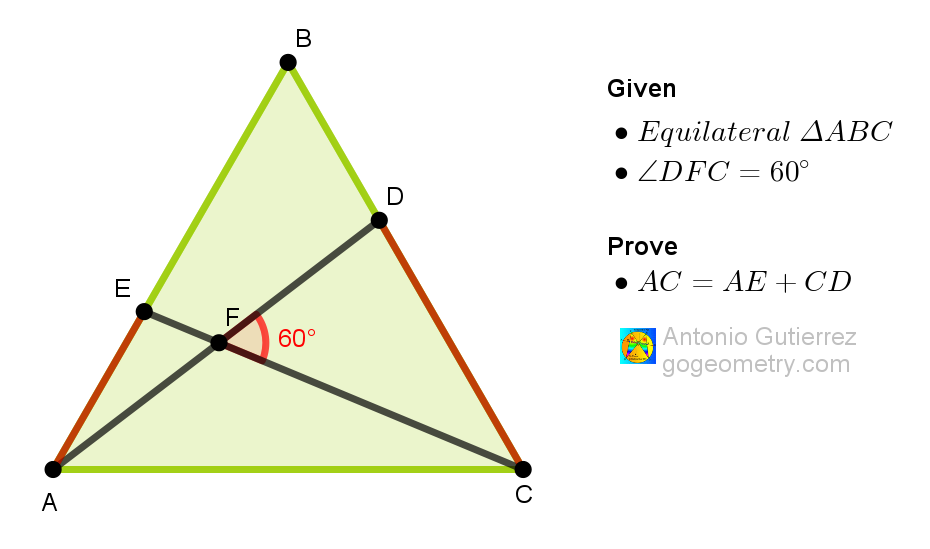
Click for additional details.
Share your solution by clicking 'Comment' below the post or entering your solution or comment in the 'Enter Comment' field and pressing 'Publish'.
Share your solution by clicking 'Comment' below the post or entering your solution or comment in the 'Enter Comment' field and pressing 'Publish'.
We show that triangles ADC and BEC are congruent.
ReplyDeleteIndeed, the quadrilateral BEFD is concyclic because <EBD = external <DFC = 60º, then also <BEF = external <FDC = <ADC.
So triangles ADC and BEC have two equal angles <EBC = EBD = 60º = <ACD, and <ADC = <FDC = <BEF = <BEC. Also these triangles have congruent side AC = BC, both opposite to the equal angles <ADC = <BEC (then congruence by ASA). Observe that AD = EC, both opposite to the 60º angles, and finally CD = BE.
But AE + EB = AB = AC and CD = BE = EB implies AC = AE + CD, as desired.
Joaquim Maia
Rio de Janeiro
Brazil
BEFD is concyclic since < AFD = 60 = < B
ReplyDeleteSo < BEC = < ADC
Hence Triangles ADC & BEC are congruent ASA
Therefore AE = BD = BC - CD = AC - CD from which
AE + CD = AC
Sumith Peiris
Moratuwa
Sri Lanka
TrAEC,TrADB are congruent (AC=AC, <B=<B, <ACE=<BAD=<60°- <FAC) so
ReplyDeleteAE=BD thus AE+CD=BD+CD=BC=AC
Georgios Kousinioris
Gastouni
Greece
TrAEC,TrADB are congruent (AC=AB, <A=<B, <ACE=<BAD=<60°-<FAC) so
ReplyDeleteAE=BD
Therefore AE+CD=BD+CD=BC=AC
Georgios Kousinioris
Gastouni
Greece