Friday, June 9, 2023

Geometry Problem 1543: Calculating the Area of Quadrilateral ABED in a Square with a Side Length of 20 and an Intersecting Arc

Geometry Problem 1543. Post your solution in the comment box below.
Level: Mathematics Education, K-12 School, Honors Geometry, College.

Get the full picture! Click below to uncover the details.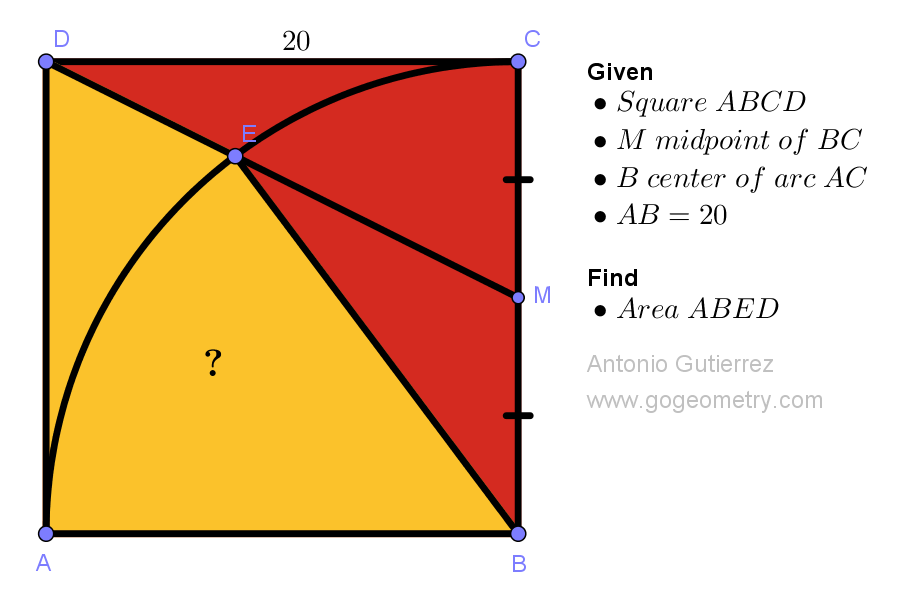

Geometry Problem 1543: Calculating the Area of Quadrilateral ABED in a Square with a Side Length of 20 and an Intersecting Arc

Click for additional details.

Share your solution by clicking 'Comment' below the post or entering your solution or comment in the 'Enter Comment' field and pressing 'Publish'.

Posted by Antonio Gutierrez at 4:27 PM
Labels: , , , , , ,

7 comments:

  1. AnonymousJune 10, 2023 at 6:52 AM

    240 using coordinate geometry

    ReplyDelete
  2. Sumith PeirisJune 13, 2023 at 12:10 AM

    Geometry Solution

    Extend DM to meet AB extended at N. Draw a perpendicular EF from E to meet AB at F. Let DE = p and AF = t.

    Triangles MCD & MBN are congruent and BN = 20. AN is a diameter of the semicircle AECN and p = 4V5
    t^2 + (2t)^2 = (2p)^2 = 320 => t = 8

    Therefore S(ABED) = S(AED) + S(AEB) = 1/2 X p X 2p+ 1/2 X 2t X 20
    = p^2 + 20t = 80 + 160 = 240

    Sumith Peiris
    Moratuwa
    Sri Lanka

    ReplyDelete
    Replies
    1. Gewürtztraminer68January 5, 2024 at 2:57 AM

      I would be grateful for more detailed explanations of your demonstration

      Delete
    2. Sumith PeirisJanuary 11, 2024 at 2:55 AM

      what is the step you cannot understand? do let me know

      Delete
  3. AnonymousJune 25, 2023 at 12:19 PM

    Find formula of circle Centre (20,0)
    Find formula of line DM
    Use to find coordinates of crossing point with the arc
    (8,16)
    Divide the yellow area into 2 triangles and 1 rectangle
    6x16=96 8x16=128 4x4=16
    Add together to make 240

    ReplyDelete
  4. Sumith PeirisJanuary 6, 2024 at 3:29 AM

    Trigonometry Solution

    Extend BEM to meet AD extended at N
    < AEN = 90 as I showed before
    If < MBC = @ then we can show that < EDM = 90 - @

    So S(DEM) = 1/2 X 20 X 10 X sin (90-2@) = 100.cos 2@
    = (1-tan^2 @)/(1 + tan^2 @) = (1 - (1/2)^2) / (1 + (1/2)^2) = 60

    So S(ABED) = S(ABCD) - S(BCM) - S(DEM)
    = 400 - 100 - 60 = 240

    Sumith Peiris
    Moratuwa
    Sri Lanka

    ReplyDelete
  5. Sumith PeirisJanuary 7, 2024 at 4:09 AM

    2nd Geometry Solution
    Extend DM to meet AB extended at N. Complete Rectangle EXBY, X on AB & Y on CM

    As before < AEM = 90

    So considering Triangle ADN,
    AE = 20 X 40 / 20V5 = 8V5 and so
    DE = 4V5 by using Pythagoras on Triangle ADE and EM = 10V5 - 4V5 = 6V5
    Hence MY = 6 and EX = 16
    So S(AEB) = 20 X 16 /2 = 160
    S(ADE) = 8V5 X 4V5 /2 = 80
    Adding S(ABED) = 160+80 = 240

    Sumith Peiris
    Moratuwa
    Sri Lanka

    ReplyDelete

Subscribe to: Post Comments (Atom)