Geometry Problem 1540. Post your solution in the comment box below.
Level: Mathematics Education, K-12 School, Honors Geometry, College.
Get the full picture! Click below to uncover the details.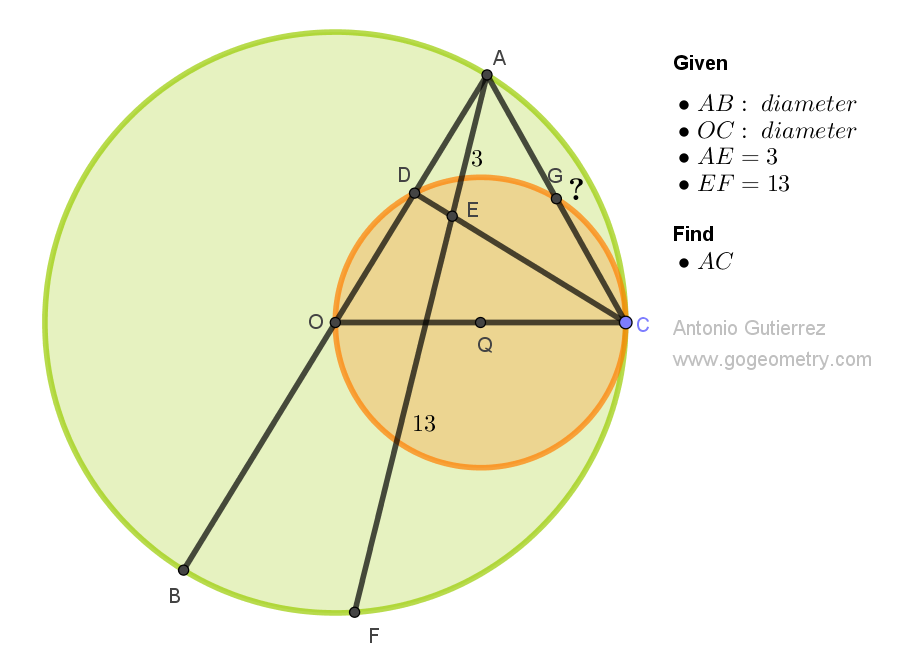
Click for additional details.
Share your solution by clicking 'Comment' below the post or entering your solution or comment in the 'Enter Comment' field and pressing 'Publish'.
Share your solution by clicking 'Comment' below the post or entering your solution or comment in the 'Enter Comment' field and pressing 'Publish'.
Draw the common tangent XCY at C
ReplyDeleteOG & BC are both perpendicular to AC, so OG//BC
<AFC = B = <XCY = B = <GOC = <GOA = <ACE
Hence AC is a tangent to circle FEC at C
So AE. AF = AC^2
Therefore AC^2 = 3 X 16
AC = V48 = 4V3
Sumith Peiris
Moratuwa
Sri Lanka
https://photos.app.goo.gl/wYT97Ju9ijb1Ww9a6
ReplyDeleteConnect OG and FC
Since AB= 2OC => G is the midpoint of AC and OG is the bisector of angle AOC
We have ^(AFC)=^(AOG)=^(ACD)= u
Triangle AEC similar to ACF ( case AA)
So AE/AC=AC/AF
AC^2=AC.AF= 48 and AC= 4.sqrt(3)
We can use the solution of triangles take ∠BAC = θ , ∠AED = ɣ, AC = x and radius = r;
ReplyDeletethen by property of circle ∠BOC = 2θ
Apply sine rule in ▵OAC which is an isosceles triangle,
sin(2θ)/x=sin(θ)/r
apply sine rule in ▵BAF;
sin(ɣ)/16=1/2r
apply sine rule in ▵DAE;
sin(ɣ)/(r/2)=1/3
∴sin(ɣ)=r/2
∴r=√(48)
and after further calculations θ=60°
∴x=√(48)=4√3
This comment has been removed by the author.
ReplyDelete