Saturday, March 11, 2023

Geometry Problem 1525: Unveiling the Secrets of an Equilateral Triangle in Right Triangle Geometry: Finding the Midpoint Distance between Segments. Difficulty Level: High School.

Geometry Problem 1525. Post your solution in the comment box below.
Level: Mathematics Education, K-12 School, Honors Geometry, College.

Details: Click on the figure below.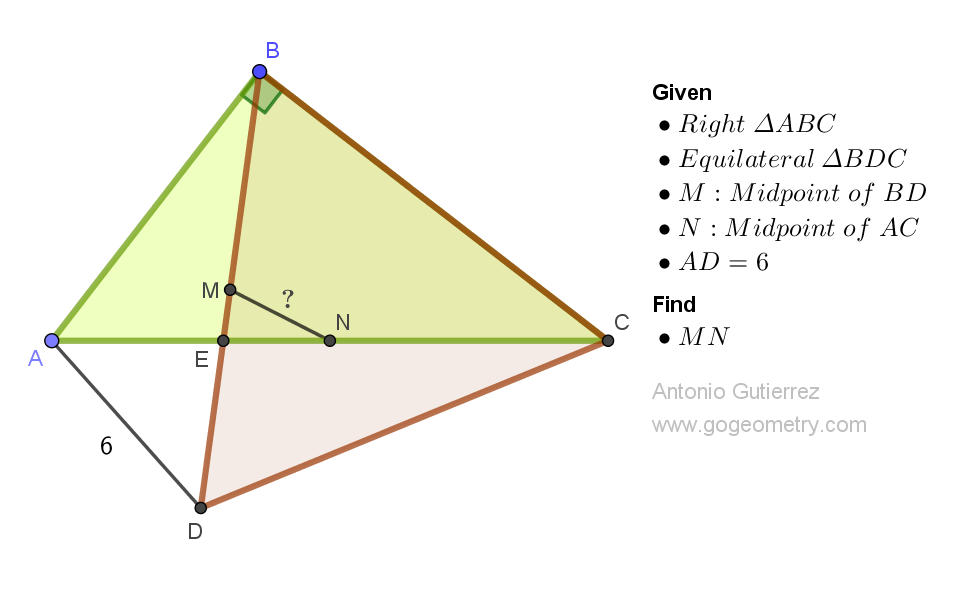

Geometry Problem 1525: Unveiling the Secrets of an Equilateral Triangle in Right Triangle Geometry: Finding the Midpoint Distance between Segments. Difficulty Level: High School.

More Details

To post a solution to this problem click Comment underneath the post, or click into the line that says, “Enter Comment.” Type what you want to say and press Publish to post your solution.

Posted by Antonio Gutierrez at 1:00 PM
Labels: , , , , , ,

5 comments:

  1. c.t.e.o.March 11, 2023 at 4:08 PM

    MN = 3

    ReplyDelete
    Replies
    1. c.t.e.o.March 12, 2023 at 11:36 AM

      https://photos.app.goo.gl/Qt4wWUDARG6fzTrbA

      Delete
    2. c.t.e.o.March 15, 2023 at 5:02 PM

      Why the sketch is deleted

      Delete
    3. Antonio GutierrezMarch 16, 2023 at 2:14 PM

      I'm sorry to hear that your sketch was deleted from the blog. there may have been a technical issue that led to the deletion. I have just re-sent your post to the blog. Thank you.

      Delete
  2. Sumith PeirisMarch 11, 2023 at 11:03 PM

    Let P be the mid-point of CD

    In Triangles BMN & CPN,
    < MBN = < PCN (= A - 30)
    BN = NC (since N is the circumcenter of right triangle ABC)
    BM = MP (since Triangle DPM is equilateral)
    So Triangles BMN & CPN are congruent SAS

    Now using the midpoint theorem in Triangle ACD,
    MN = NP = AD/2 = 6/2 = 3

    Sumith Peiris
    Moratuwa
    Sri Lanka

    ReplyDelete

Subscribe to: Post Comments (Atom)