Tuesday, March 28, 2023

Geometry Problem 1530: Unlock the Secrets of Geometric Angles: Calculate the Measurement of an Angle in a Square and Rectangle Figure Today

Geometry Problem 1530. Post your solution in the comment box below.
Level: Mathematics Education, K-12 School, Honors Geometry, College.

Details: Click on the figure below.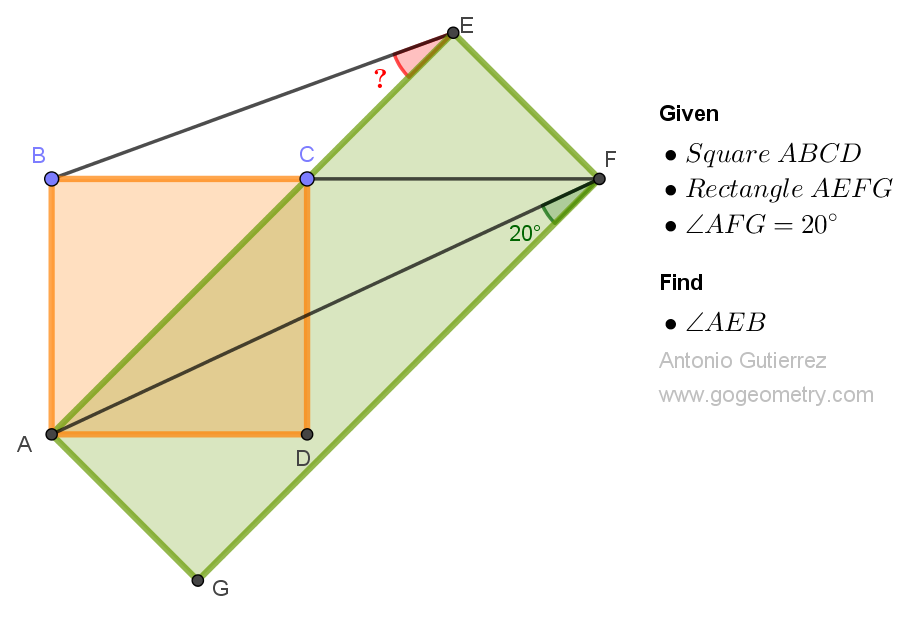

Geometry Problem 1530: Unlock the Secrets of Geometric Angles: Calculate the Measurement of an Angle in a Square and Rectangle Figure Today.

More Details

To post a solution to this problem click Comment underneath the post, or click into the line that says, “Enter Comment.” Type what you want to say and press Publish to post your solution.

Posted by Antonio Gutierrez at 5:19 PM
Labels: , , , , ,

6 comments:

  1. Sumith PeirisMarch 28, 2023 at 7:49 PM

    ABEF is a circle with AF as diameter.
    So < BFE = < BAE = 45 and so
    < AFB = 90 - 45 - 20 = 25

    Therefore < AEB = < AFB = 25

    Sumith Peiris
    Moratuwa
    Sri Lanka

    ReplyDelete
  2. AnonymousMarch 28, 2023 at 10:17 PM

    25°

    ABEF is a cyclic quadrilateral
    (20+45)+(90+AEB)=180
    AEB=25

    ReplyDelete
  3. c.t.e.o.March 29, 2023 at 5:17 AM

    x=25° : https://photos.app.goo.gl/d7LNrJchKUm4RTG66

    ReplyDelete
  4. Peter TranMarch 29, 2023 at 9:49 AM

    ABEF is a cyclic quadrilateral so ^(BEA)=^(BFA)
    ABCD is a square => ^(BCA)=^(ECF)=^(EFC)= 45
    so ^(BFA)=^(BEA)=90-45-20=25

    ReplyDelete
  5. Pradyumna AgasheMarch 29, 2023 at 10:38 AM

    Angle ABF = Angle AEF = 90 degrees, hence Quadrilateral ABEF is cyclic with AF as diameter.
    Angle BAE= Angle BFE =45 deg.
    Angle AFB= 90 - 45 - 20 = 25 deg.
    Since ABEF is cyclic Angle AEB = Angle AFB = 25 degrees.

    ReplyDelete
  6. AnonymousJuly 23, 2023 at 1:17 AM

    25

    ReplyDelete

Subscribe to: Post Comments (Atom)