Geometry Problem 1516. Post your solution in the comment box below.
Level: Mathematics Education, K-12 School, Honors Geometry, College.
Details: Click on the figure below.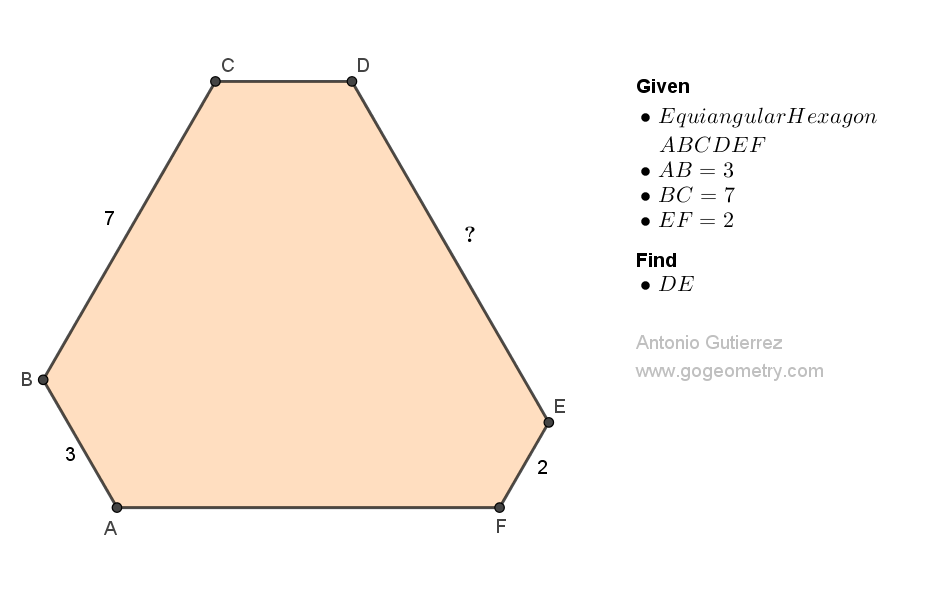
More Details
To post a solution to this problem click Comment underneath the post, or click into the line that says, “Enter Comment.” Type what you want to say and press Publish to post your solution.
To post a solution to this problem click Comment underneath the post, or click into the line that says, “Enter Comment.” Type what you want to say and press Publish to post your solution.
https://photos.app.goo.gl/Gv5Rwt8cf4DriGjp6
ReplyDeleteForm equilateral Triangle LMN, L on AB extended, M on CD extended and N on EF extended. Let AF = NF = x and DE = EM = y
ReplyDeleteSince LN = MN,
x + 7 + 3 = x + 2 + y from which y = 8 = DE
Sumith Peiris
Moratuwa
Sri Lanka
Solution 2
ReplyDeleteIt is easy to see that CD//AF and BC//EF.
Complete parallelogram with sides CDM, MEF, FAT, TBC
So TC = FM and so 7 + 3 = 2 + DE since Tr.s ABT and DEM are both equilateral
Hence DE = 8
Sumith Peiris
Moratuwa
Sri Lanka