Geometry Problem 1500. Post your solution in the comment box below.
Level: Mathematics Education, K-12 School, Honors Geometry, College.
Details: Click on the figure below.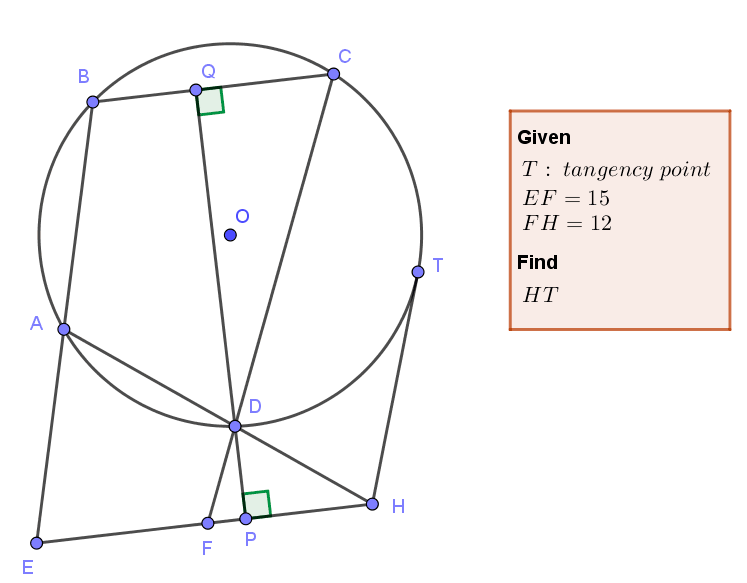
More Details
To post a solution to this problem click Comment underneath the post, or click into the line that says, “Enter Comment.” Type what you want to say and press Publish to post your solution.
To post a solution to this problem click Comment underneath the post, or click into the line that says, “Enter Comment.” Type what you want to say and press Publish to post your solution.
Since ABCD is cyclic quadrilateral, Angle EAD = Angle BCD
ReplyDeleteAnd BC is parallel to EH hence Angle EFD= 180 - Angle BCD.
Since we have Angle EAD + Angle EFD=180, quadrilateral AEFD is cyclic.
We get HT^2=HD×HA=HF×HE,
HT^2=12×(12+15)=324
HT=18.
Since, BC//EH, ABCD cyclic quadrilateral, One can easily prove that ADFE is also cyclic quadrilateral. (Angle ADC=Angle AEH). Thus, HT^2 = AH x DH=EH x FH=27 x 12=324. So, HT = 18.
ReplyDelete