Geometry Problem 1498. Post your solution in the comment box below.
Level: Mathematics Education, K-12 School, Honors Geometry, College.
Details: Click on the figure below.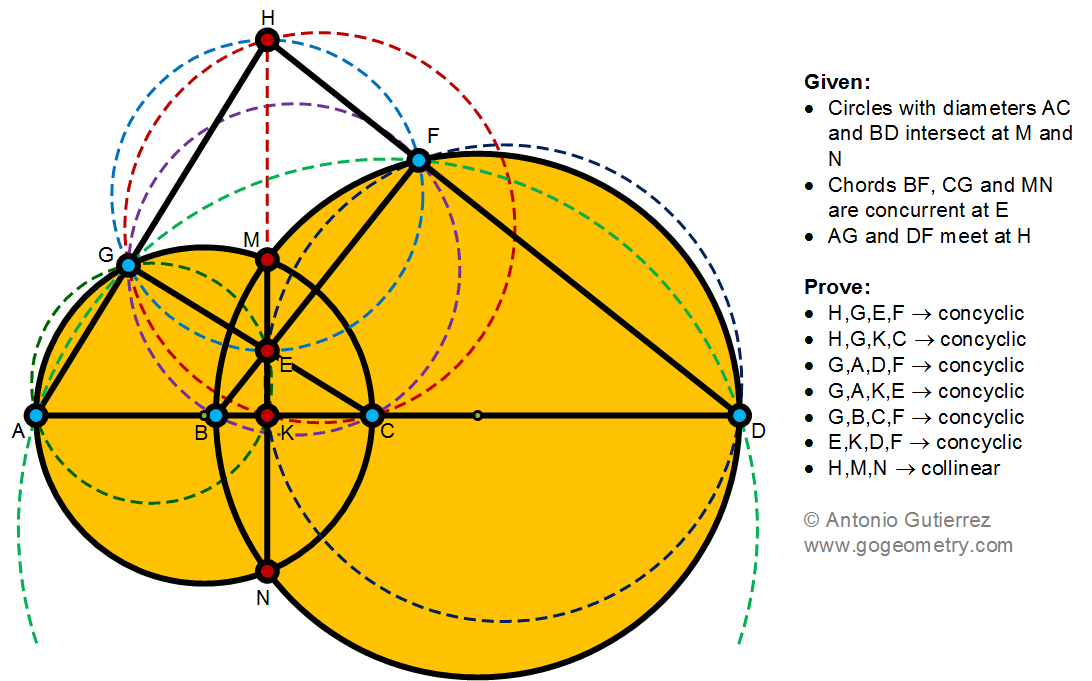
More Details
To post a solution to this problem click Comment underneath the post, or click into the line that says, “Enter Comment.” Type what you want to say and press Publish to post your solution.
To post a solution to this problem click Comment underneath the post, or click into the line that says, “Enter Comment.” Type what you want to say and press Publish to post your solution.
Since E is on the radical axis of circles [ACG] and [BDF]
ReplyDeleteSo we have ẸC.EG=EB.EF= power of point E to both circles….(1)
Let MN meet AG at H’ and MN meet DF at H
Power of E with circle [H’GKC]= EG.EC= EK.EH’… (2)
Power of E with circle [HFKB] =EB.EF=EK.EH….. (3)
Compare (1),(2) and (3) => EH=EH’ => H coincide to H’
And H, M, N are collinear
this result will help to prove the questions 1 to 6