Geometry Problem 1547. Post your solution in the comment box below.
Level: Mathematics Education, K-12 School, Honors Geometry, College.
Get the full picture! Click below to uncover the details.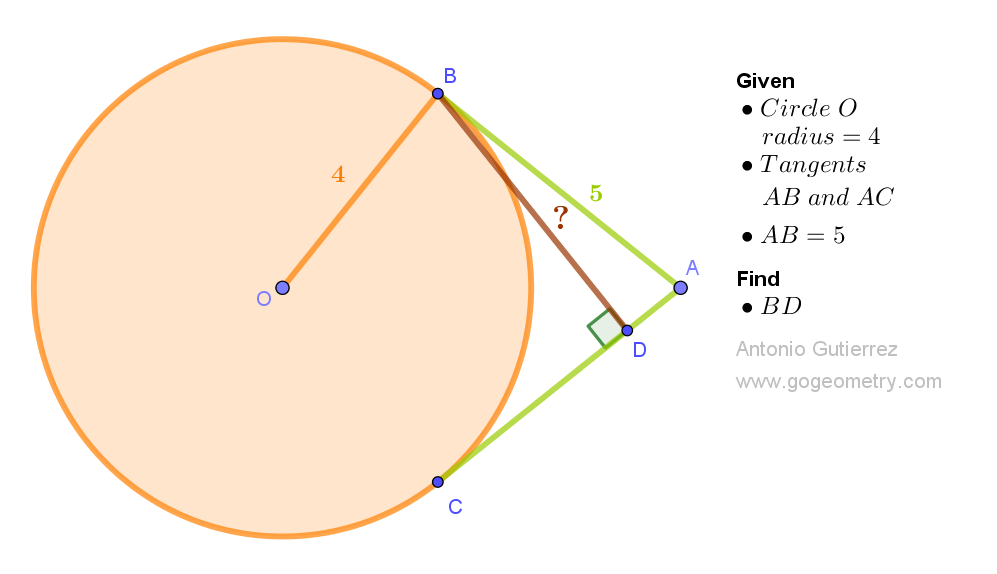
Click for additional details.
Share your solution by clicking 'Comment' below the post or entering your solution or comment in the 'Enter Comment' field and pressing 'Publish'.
Share your solution by clicking 'Comment' below the post or entering your solution or comment in the 'Enter Comment' field and pressing 'Publish'.
Let BD cut the circle at E. Let F be the midpoint of BE. Let BF = y and OF = z
ReplyDeleteOFDC is a Rectangle. Triangles OBF & ABD are similar
So 4/5 = y/(5-z) = z/(y+4) which gives z = 4(y+4)/5
Substituting for z, y/(5 - 4(y+4)/5)) = 4/5
Simplifying y = 36/41
Therefore BD = 4 + 36/41 = 4.89 approximately
Sumith Peiris
Moratuwa
Sri Lanka
From right triangle OBA, tan(angleBAO) = 4/5, so BAO = atan(4/5)
ReplyDeleteNow, BAC = 2 BAO and from right triangle BDA,
BD = 5 sin(BAC) = 5 sin(2*atan(4/5) = 4.878
Christos