Geometry Problem 1532. Post your solution in the comment box below.
Level: Mathematics Education, K-12 School, Honors Geometry, College.
Details: Click on the figure below.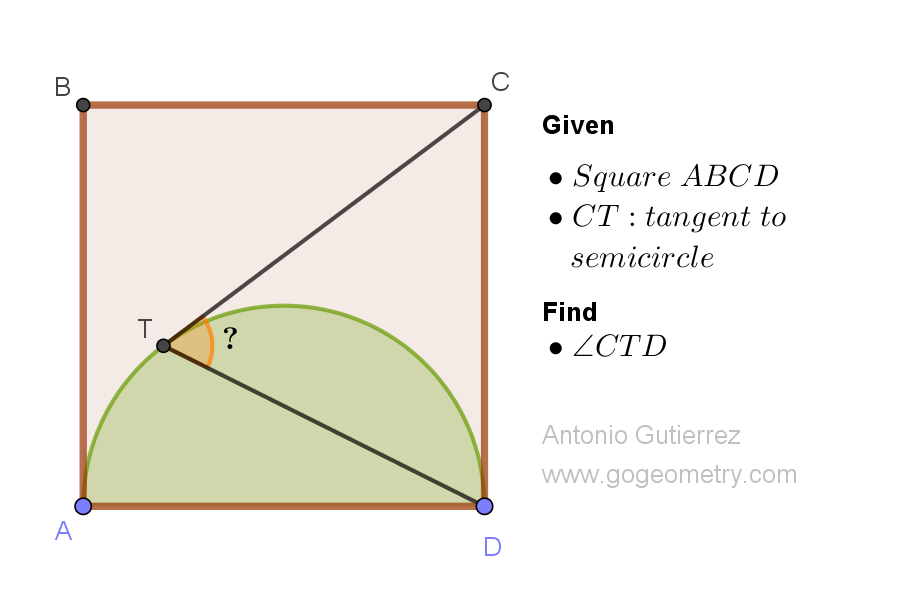
More Details
To post a solution to this problem click Comment underneath the post, or click into the line that says, “Enter Comment.” Type what you want to say and press Publish to post your solution.
To post a solution to this problem click Comment underneath the post, or click into the line that says, “Enter Comment.” Type what you want to say and press Publish to post your solution.
Let M is the midpoint of AD
ReplyDeletesince TD ⊥MC => ∡ (CTD)=∡ (CDT)=∡ (CMD)
∡ (CMD)= atan(CD/MD) =atan(2)~63.43 degrees
Let O be the mid point of AD.
ReplyDeleteThen OTCD is a kite and < TCO = < DCO = arctan (1/2).
So ? = 90 - arctan (1/2)
90 - arctan (1/2) is the same as arctan(2) = 63.4 degrees approximately
DeleteBT/AT = sqrt2 is another result from this problem
ReplyDeleteAlso BT bisects the Right Angle ATD
Delete